1. Obtain the expression for the magnetic field on the axis of a finite solenoid carrying n turns per unit length.

The turns of the coil are shown below with a dot indicating that the current is coming out of the page and a cross indicating that it is going into the page. Consider a point P on the axis of the solenoid.

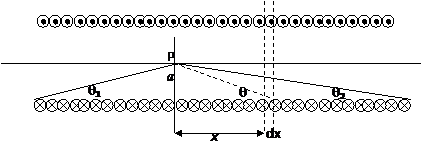
Let a be the radius of the solenoid. Consider a length dx at a distance x from P. The amount of  current that this section contains is 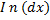. This section can be considered as a circular loop of radius a, the field due to which at P is given by

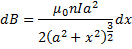
Let 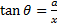, so that 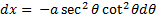.  The denominator of the above expression is given by

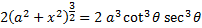
The field due to the solenoid at P is given by

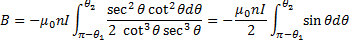
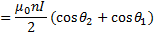
As the length of the solenoid approaches infinity both the angles 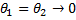 and we get back  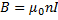as expected.

2. A rectangular loop  of dimension  carries a current . A  long wire carrying a current  is at a distance  from one of the sides of length  it, the rectangle and the wire are in the same plane.  The direction of current in the wire and that flowing in the nearer side of the rectangle are parallel. Calculate the force on the loop.

The force on the two shorter sides cancel because they carry current in the opposite direction and the force on each current element on one of the sides is canceled by the force on the corresponding element on the other side. The force of attraction on the nearer side is 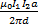and  the repulsive force on the far side is 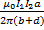. Thus the net force is attractive and is given by

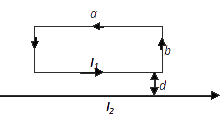
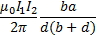
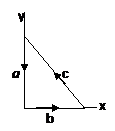
3. A single current loop is in the shape of a right triangle of sides a, b, c.  A uniform magnetic field of strength 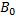 is directed  parallel to the hypotenuse. Calculate the total force on the loop as well as the torque acting on the loop.

Let the triangle be in the xy plane. The unit vector along c is 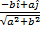. Thus,

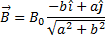
Force on side c is zero. Force on side a is 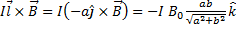. It can be net force is zero. This is also seen by adding up the force vectoriall since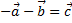.  The torque is not zero however. As the torque can                    

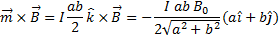
4. A semicircular loop of radius R lies in the xy plane carrying a current I in the counterclockwise direction. Magnetic field B points perpendicular to the diameter (along the y direction). Calculate the force on the straight-line segment as well as on the semi circular arc.

The force on the straight-line segment is .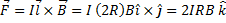

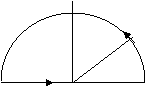
on the length element is

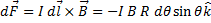
Integrating, the force on the arc is

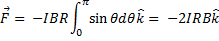
(Of course, the net force on the loop has to be zero!